In Singapore, students only start to do speed, distance, time practice problems in Primary 6.
A few years ago, speed ratio was the most challenging type of question to be tested. Thus, I included that in the Word Problems On Speed Boom deck resource.

In this blog post, I will go through 3 selected speed distance time questions.
Before I show you the speed distance time word problems with solutions, let me explain what speed ratio is.
Speed Ratio Concepts
When discussing speed ratios, there are two types.
One involves the straightforward use of a ratio to solve the question.
The other involves the concept that speed is directly proportional to distance and inversely proportional to time (note that these terms are not taught in Primary 6).
Here are some clues to identify this type of question:
- Lack of information – do not have 2 out of 3 of the units (speed, distance, time).
- Either the same distance or the same time is taken
- Often, the difference or total is provided.
The logic behind this is that when the speed is faster, the time taken will be shorter, resulting in smaller units.
Distance Ratio
In this question, the distance remains the same for each boy, as each needs to run 100 m.
The distance ratio:
| Gary | : | Carl | : | Zack | |
|---|---|---|---|---|---|
| 100 | : | 100 – 20 | : | 100 – 40 | |
| 100 | : | 80 | : | 60 | |
| X1.25 to make Carl 100. | 125 | : | 100 | : | 75 |
Thus, Zack had run 75 m by the time Carl reached the finishing point (100 m).
Speed and Distance Ratio : Directly Proportional
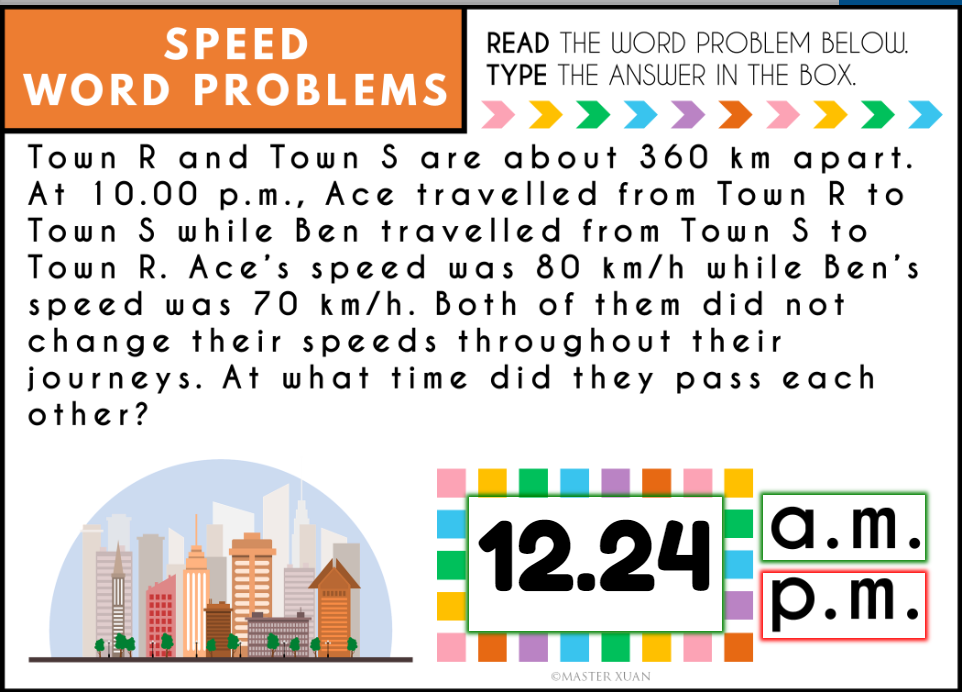
| Ace | : | Ben | |
| Speed ratio | 80 | : | 70 |
| Distance ratio | 80 | : | 70 |
Total units of distance traveled by Ace and Ben → 80 u + 70 u = 150 u
150 u → 360 km
10 u → 360 km ÷ 15 = 24 km
80 u → 24 km x 8 = 192 km
Time taken for Ace to pass Ben -> Distance ÷ Speed = 192 km ÷ 80 km/h = 2.4 h = 2 h 24 min
After 2 hours and 24 minutes from 10:00 p.m. it is 12:24 a.m.
Currently, I no longer use the speed ratio method to solve this type of question. Instead, we use the formula of time taken to meet up to solve it.
Speed and Time Ratio: Inversely Proportional

Since Ryan is using the same route, distance remains unchanged.
| House to Park | : | Park to House | |
| Speed ratio | 80 | : | 100 |
| Time ratio | 100 | : | 80 |
The difference in time taken with the change in speed → 24 min – 8 min = 16 min
The difference in time taken (units) with the change in speed → 100 u – 80 u = 20 u
20 u → 16 min
100 u → 16 min x 5 = 80 min
Distance traveled → speed x time = 80 m/min x 80 min = 6 400 m = 6.4 km
Final Thoughts
Do you find these speed distance time practice problems with solutions useful? These questions come from the resource Word Problems On Speed in my TPT store.
Even if these types of questions are no longer common in primary level, these questions can still be useful in secondary level.
Even if these types of questions are no longer common at the primary level, they can still be useful in secondary education.
If you need more speed distance time questions and a variety of resources for your students to practice, consider buying the Speed Distance Time Activities Mega Bundle at a discounted price!
Related Read: 5 Easy And Useful Resources To Teach Speed, Distance, Time
Here are some pins that you can save to bookmark this post. Save them to your Pinterest Board now! ↓