As of 2023, in Singapore, students are first introduced to percentage concepts in Primary 5.
Students need to understand what percentage is, and learn how to convert percentage to fractions or decimals and vice versa. After learning the basics of the percentage part of a whole, it is time to move on to word problems.
Understanding and solving percentage word problems is crucial, as it allows students to apply their knowledge in real-world scenarios. To make this process more manageable, I have categorized these percentage word problems into 18 common question types.
In this blog post, I will share an example of the 18 percentage word problems that are commonly tested for Primary 5 students. The solutions are more detailed than those in the Percentage Word Problems Practice Worksheets.
So if your students need more explanation or detailed solutions, share with them this blog post!
These percentage concepts not only help them ace their math classes but also equip them with valuable skills that are applicable in real-life situations.
So, if you want more practice, buy the Percentage Word Problems Practice Worksheets in my TPT store!

Percentage Part Of A Whole
There are 50 candies in a box.
18 of them are sweet.
What percentage of the candies are sweet?
Solution
First, write the fraction. The noun after the keyword “of” will be the denominator.
Then, multiply by 100% to convert the fraction to percentage.
18/50 x 100% = 36%
Percentage After Change
A group of 100 pupils went on an excursion.
60 of them were girls.
If another 50 boys joined the excursion, what percentage of the pupils were boys in the end?
Solution
Numbers of boys at first → 100 – 60 = 40
Numbers of boys in the end → 40 + 50 = 90
Total numbers of pupils → 100 + 50 = 150
Percentage of the pupils were boys in the end → 90/150 x 100 = 60%

Finding A Part (I)
A school has 600 students.
30% of the students walk to school.
How many students walk to school?
Solution
Method 1:
Number of students walk to school → 30/100 x 600 = 180
Method 2:
100% → 600
1% → 6
30% → 6 x 30 = 180
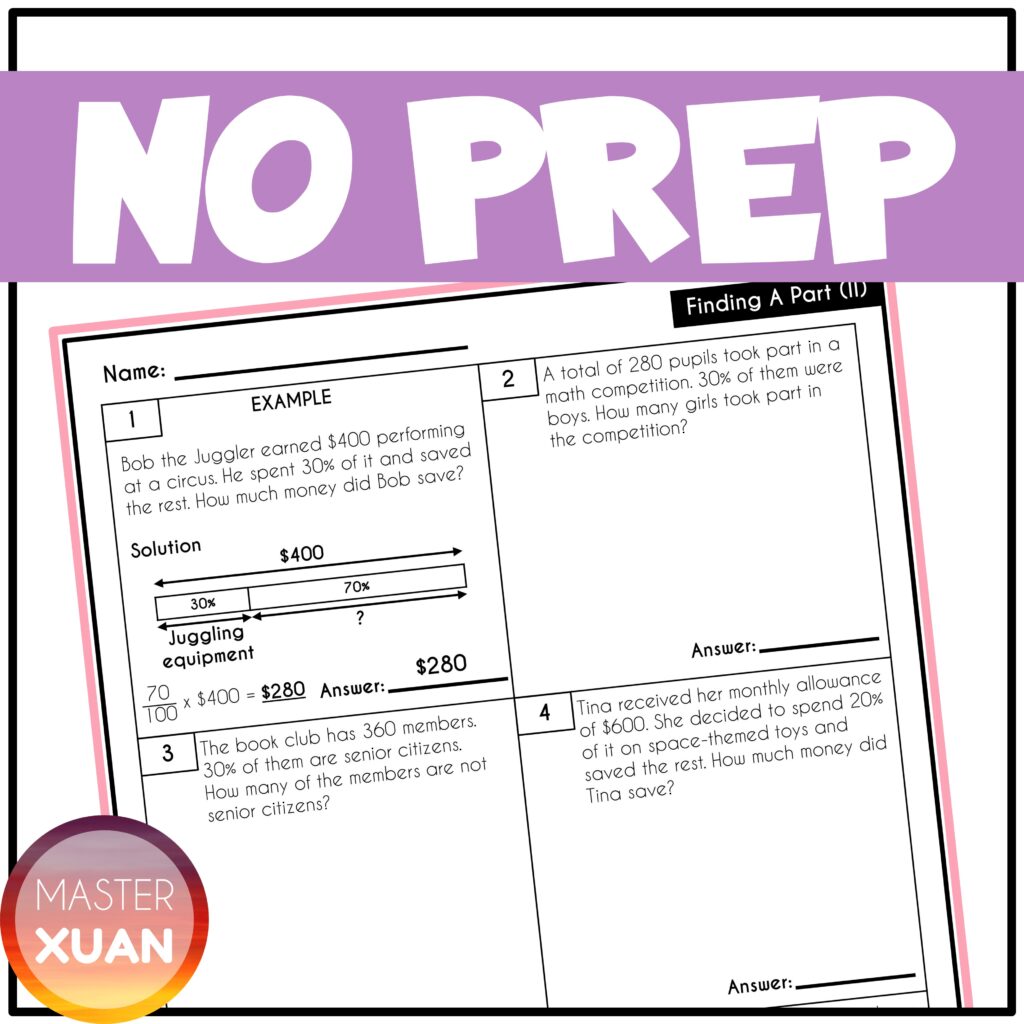
Finding A Part (II)
Bob the Juggler earned $400 performing at a circus.
He spent 30% of it and saved the rest.
How much money did Bob save?
Solution
Method 1:
Percentage saved → 100% – 30% = 70%
Amount of money Bob saved → 70/100 x $400 = $280
Method 2:
100% → $400
1% → $4
70% → $4 x 70 = $280
Finding A Part (III)
Fann spent 20% of her money and had $320 left.
How much money did she spend?
Solution
Percentage Left → 100% – 20% = 80%
80% → $320
1% → $320 ÷ 80 = $4
20% → 20 x $4 = $80
Finding A Part (IV)
At a bakery, there are 200 cupcakes.
70% of them are vanilla, and the rest are chocolate.
80% of the chocolate cupcakes have sprinkles.
How many chocolate cupcakes are without sprinkles?
Solution
Percentage of chocolate cupcakes → 100 – 70 = 30
Numbers of chocolate cupcakes → 30/100 x 200 = 60
Percentage of chocolate cupcakes without sprinkles → 100% – 80% = 20%
20% → 20/100 x 60 = 12
Finding The Whole
Bobby spent 10% of his allowance on a giant lollipop.
The lollipop cost $2.50.
How much money did Bobby have at first?
Solution
10% → $2.50
1% → $2.50 ÷ 10 = $0.25
100% → $0.25 x 100 = $25
Find The Interest
Olivia deposited $6 000 in a savings account for one year.
The interest rate is 4% per year.
How much interest will she earn after one year?
Solution
Method 1:
Interest → 4/100 x $6 000 = $240
Method 2:
100% → $6 000
1% → $6 000 ÷ 100 = $60
4% → $60 x 4 = $240
Find The Price After Interest
Mr. Johnson deposits $6 500 in a bank for one year.
The interest rate is 3% per year.
What is the total amount of money he will have in the bank at the end of one year?
Solution
Method 1:
Interest → 3/100 x $6 500 = $195
Amount of money at the end of one year → $6 500 + $195 = $6 695
Method 2:
Amount of money at the end of one year → 103/100 x $6 500 = $6 695
Method 3:
100% → $6 500
1% → $6 500 ÷ 100 = $65
103% → $65 x 103 = $6 695
Find The Discount
The usual price of a magic broom was $125.
At a sale, it was sold at a discount of 8%.
How much was the discount?
Solution
Method 1:
Discount → 8/100 x $125 = $10
Method 2:
100% → $125
1% → $125 ÷ 100 = $1.25
8% → $1.25 x 8 = $10
Find The Price After Discount
A potion kit was originally priced at $60.
Alex bought it with a 25% discount.
How much did Alex pay for the potion kit?
Solution
Method 1:
Discount → 25/100 x $60 = $15
Discounted Price → $60 – $15 = $45
Method 2:
Discounted price percentage → 100% – 25% = 75%
Discounted Price → 75/100 x $60 = $45
Method 3:
Discounted price percentage → 100% – 25% = 75%
100% → $60
1% → $60 ÷ 100 = $0.60
75% → $0.60 x 75 = $45
Find The Price Before Discount
After a 25% discount, the cost of an enchanted necklace is $225.
What was the original price of the necklace?
Solution
Discounted price percentage → 100% – 25% = 75%
75% → $225
1% → $225 ÷ 75 = $3
100% → $3 x 100 = $300
Find The GST
A laptop costs $800 before a 9% GST.
How much is the GST?
Solution
Method 1:
9/100 x $800 = $72
Method 2:
100% → $800
1% → $800 ÷ 100 = $8
9% → $8 x 9 = $72
Find The Price After GST
The cost of a bicycle before GST was $450.
What was the price of the bicycle including 9% GST?
Solution
Method 1:
GST → 9/100 x $450 = $40.50
Price of the bicycle including 9% GST → $450 + $40.50 = $490.50
Method 2:
Percentage of the cost of bicycle including 9% GST → 100% + 9% = 109%
Price of the bicycle including 9% GST → 109/100 x $450 = $490.50
Method 3:
100% → $450
1% → $450 ÷ 100 = $4.50
Percentage of the cost of bicycle including 9% GST → 100% + 9% = 109%
109% → $4.50 x 109 = $490.50
Find The Price Before GST
The price of a television, including 9% GST, is $654.
What is the cost of the television before GST?
Solution
The price of a television, including 9% GST → 100% + 9% = 109%
109% → $654
1% → $654 ÷ 109 = $6
100% → $6 x 100 = $600
Find The GST After Discount
The price of a bicycle was $380 before GST.
Harry bought it at a 15% discount and paid 9% GST on the discounted price.
How much was the GST?
Solution
Method 1:
Discounted price percentage → 100% – 15% = 85%
Discounted price → 85/100 x $380 = $323
GST → 9/100 x $323 = $29.07
Method 2:
100% of original price → $380
Discounted price percentage → 100% – 15% = 85%
1% of original price → $380 ÷ 100 = $3.80
85% of original price = 100% of discounted price → $3.80 x 85 = $323
1% of discounted price → $323 ÷ 100 = $3.23
9% of discounted price → $3.23 x 9 = $29.07
Find The Price After Discount And GST
Ms. Enchantress purchased a wizard’s hat which cost $80.
During a special sale, a 20% discount was given.
Additionally, she had to pay 9% GST.
How much did she pay for the wizard’s hat?
Solution
Method 1:
Discounted price percentage → 100% – 20% = 80%
Discounted price → 80/100 x $80 = $64
Price after GST → 109/100 x $64 = $69.76
Method 2:
Discounted price percentage → 100% – 20% = 80%
1% of original price → $80 ÷ 100 = $0.80
80% of original price = 100% of discounted price → $0.80 x 80 = $64
1% of discounted price → $64 ÷ 100 = $0.64
109% of discounted price → $0.64 x 109 = $69.76
Find The Price Before Discount And GST
A toy car costs $92.65 after a 15% discount and 9% GST are applied.
What was the original price of the toy car?
Solution
The price of a toy car, including 9% GST → 100% + 9% = 109%
109% of discounted price → $92.65
1% of discounted price → $92.65 ÷ 109 = $0.85
100% of discounted price → $0.85 x 100 = $85
Discounted price percentage → 100% – 15% = 85%
100% of discounted price = 85% of original price
85% of original price → $85
100% of original price → $85/85 x 100 ≈ $100
Final Thoughts

In conclusion, mastering percentage concepts is a fundamental skill for Primary 5 students in Singapore. By solving real-world word problems, these mathematical skills provide a strong foundation for both academic success and practical application.
The 18 common question types we’ve covered in this blog post serve as valuable practice exercises, offering detailed solutions to aid students in their learning journey.
These skills extend beyond the classroom, empowering students to make informed decisions in various real-life situations.
So, if you’re seeking additional practice or resources, don’t hesitate to explore the Percentage Word Problems Practice Worksheets in our TPT store.
By honing these skills, students can excel in mathematics and apply their knowledge effectively in everyday scenarios.
Related Read: 5 Ratios And Percentages Worksheets You Need To Engage Math Students