We all strive to achieve math facts fluency and to enable students to be able to reach that level of mastery, introducing compensation strategy math is one of the ways.
In this article, we’ll explore three simple ways to practice compensation strategy math that will help you teach this mental strategy with greater ease and confidence.
Understanding Compensation Strategy Math
What is Compensation Strategy Math?
Compensation strategy math is a method used to simplify mathematical calculations by adjusting numbers (adding or taking away numbers) to make them easier to work with.
This approach involves changing numbers to multiple of 10 (rounding up or down) to make the calculation simpler, then compensating for the changes made.
You can use this mental strategy for both addition and subtraction.
Example 1:
7 + 9 = 7 + 10 – 1 = 17 – 1 = 16
Example 2:
37 – 18 = 37 – 20 + 2 = 17 + 2 = 19

Why Is Compensation Strategy Math Important?
Mastering compensation strategy math is crucial because it enhances your ability to perform mental math quickly and accurately. This skill is not only useful in academic settings but also in everyday situations where quick calculations are needed.
3 Simple Ways To Practice The Compensation Strategy Math
Visual aids can help make abstract concepts more concrete and easier to understand. They can be especially helpful in compensation strategy math.
These 3 ways that I’m going to introduce to you use some visual cues.
The First Way: Ten Frames
Explanation of Ten Frames
Ten Frames are a visual tool used in early mathematics education to help students understand numbers and their relationships. A Ten Frame is a two-by-five grid where counters or dots are placed to represent numbers up to ten. This method is especially useful for teaching basic addition and subtraction.
Using Ten Frames for Compensation Strategy
When using Ten Frames to practice the compensation strategy, students can visualize how adjusting numbers affects the calculation. By moving counters between frames, they can see the impact of rounding and compensation.
Step-by-Step Guide
- Set Up the Frame: Start with 2 Ten Frames filled to represent the two addends.
- Adjust the Number: Add counters to the frame with a larger number to create a rounded number.
- Perform the Calculation: Use the simplified numbers for the calculation.
- Compensate: Adjust the final answer to reflect the initial changes. To do so, remove the same number of counters added earlier.
Example:
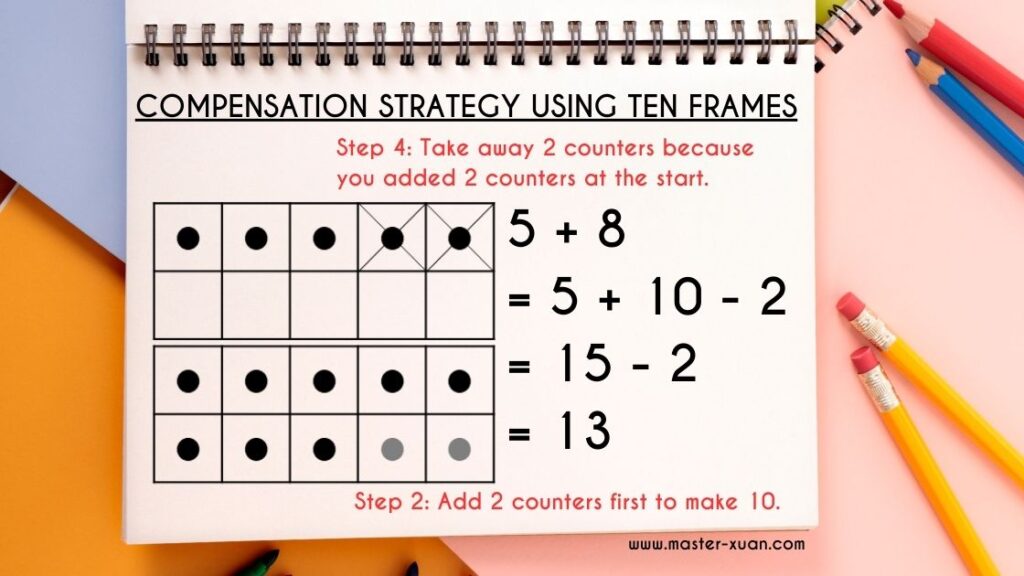
Calculate 5 + 8 using Ten Frames.
- Set Up: Place 5 counters in the first frame and 8 in the second frame.
- Adjust: Add 2 counters to the second frame to make it into 10.
- Calculate: 5 + 10 = 15.
- Compensate: Remove the 2 counters from the first frame to get the answer: 15 – 2 = 13.
The Second Way: Number Lines
Explanation of Number Lines
Number Lines are a visual representation of numbers on a straight line, often used to illustrate basic arithmetic operations. They help students understand the concept of number sequences and the effects of adding or subtracting numbers.
Using Number Lines for Compensation Strategy
Number Lines allow students to see the impact of rounding numbers and the subsequent compensation visually. By marking points and making adjustments directly on the line, they can follow the process more intuitively.
Step-by-Step Guide
- Draw the Number Line: Draw a line with or without marked intervals (e.g., 0 to 20).
- Mark the Number: Place point on the number line to represent the number with the smaller digit in the ones place.
- Adjust the Numbers: Round the addend with the larger digit in the ones place up to the nearest ten.
- Perform the Calculation: Use the rounded numbers for a simpler calculation.
- Compensate: Adjust the final result to account for the rounding.
Example 1
Calculate 65 + 27 using a number line.
- Draw the Number Line: Draw a number line from 0 to 100.
- Mark the Number: Mark 65 on the number line.
- Adjust the Numbers: Round the addend 27 to 30.
- Perform the Calculation: Visualize the addition of 30 by moving from 65 to 95.
- Compensate: Then move back 3 steps from 95 to 92 to compensate the original +3.
Example 2
Calculate 82 – 19 using a number line.
- Draw the Number Line: Draw a number line.
- Mark the Number: Mark 82 on the number line.
- Adjust the Numbers: Round the addend 19 to 20.
- Perform the Calculation: Visualize the subtraction of 20 by moving from 82 to 62.
- Compensate: Then move forward 1 step from 62 to 63 to compensate the original -1.

The Third Way: Role Playing Game
Role-playing involves students taking on specific roles and acting out scenarios that involve mathematical problems. This method can make abstract concepts more concrete and relatable.
By simulating real-life situations where compensation strategy math is applicable, students can see the relevance and practicality of what they are learning.
Role-Playing Game 1 – The Frenzy Cashier
Setup: Before starting the role-playing game, prepare cards with specific problems that students need to solve using the compensation strategy. Write the steps and answers on the back of each card.
You will need a timer.
How to Play:
- Independent Game:
- Solo Play: Students can time themselves and see how many questions they can answer correctly in 1 minute. They can aim to beat their own high score.
- Partner Game:
- Players: Player A acts as the cashier and Player B as the buyer. Player A will be the one tested.
- Gameplay: Within a set time period (20 seconds, 30 seconds, or 1 minute), Player B will flash the task cards with the question. Player A must mentally calculate and say the answer to earn a point. Player A will take the task cards as a record of the correct questions answered.
- Switch Roles: After the time is up, switch roles so Player B answers the questions. The task cards can be shuffled and reused. The player with the highest points wins.
- Class or Group Game:
- Team Setup: Each player has an equal line of students standing in front of them, each holding a task card with the question facing the player and the answer facing themselves.
- Gameplay: If the player answers correctly, the student moves to the side, allowing the player to continue answering questions held by the next student. The first player to answer all the questions wins.
Role-Playing Game 2 – The Frenzy Customer
Setup: Place price tags on different items around the room.
How to Play:
- Objective:
- Players have a set time limit (i.e. 1 minute) to collect items from the room. The goal is to collect items with a total price as close to $100 as possible.
- Winning:
- The first person to gather items nearest to $100 wins the game.
Guidelines for Role-Playing Activities
- Explain the Strategy: Before starting, ensure that all students understand the compensation strategy. Demonstrate how to adjust numbers to simplify calculations and then compensate for the adjustments.
- Encourage Thought Process: Ask students to say their thought process while solving the problems. This helps reinforce their understanding and allows them to learn from each other.
- Provide Feedback: After each role-playing session, provide feedback on how well the students applied the compensation strategy. Highlight any mistakes and explain how to correct them.
Benefits of Role-Playing in Learning Compensation Strategy Math
- Engagement: Role-playing makes learning interactive and enjoyable, which can increase student engagement and interest in math.
- Practical Application: By simulating real-life scenarios, students can see how the compensation strategy is applied outside the classroom, making the learning experience more relevant.
- Collaboration: Role-playing encourages teamwork and communication, as students work together to solve problems and discuss strategies.
Tips for Successful Role-Playing Sessions
- Start Simple: Begin with straightforward problems and gradually increase the complexity as students become more comfortable with the strategy.
- Practice Play: Try out once before officially starting so that students have a better idea how the game really works.
- Rotate Roles: Allow students to switch roles to experience different perspectives and challenges.
- Reflect and Review: After each session, take time to reflect on what was learned and review the key principles of the compensation strategy.
Role-playing is a powerful tool for teaching compensation strategy math. By engaging students in interactive scenarios, you can help them grasp the concept more effectively and see its practical applications. Encourage your students to embrace role-playing activities, and watch their understanding and confidence in math grow.
Common Mistakes and How to Avoid Them
Common Pitfalls in Compensation Strategy Math
- Over-compensating or under-compensating.
- Forgetting to adjust the final answer.
- Calculation error.
Tips for Avoiding These Mistakes
- Double-check your adjustments.
- Always compensate for changes made.
- Practice regularly to build accuracy.
Resources for Further Practice
If you’re looking for more structured practice to help your students master compensation strategy math, consider using specialized worksheets.
This Math Compensation Strategy For Addition And Subtraction Within 100 Worksheets is designed to provide step-by-step problems and detailed explanations that reinforce these essential skills.
You can find this comprehensive resource available for purchase to enhance your teaching toolkit.
Final Thoughts
Mastering the compensation strategy in math can simplify complex calculations and improve your students’ overall mathematical abilities.
By practicing with Ten Frames, Number Lines, and role playing games, your students can enhance their problem-solving skills and build confidence in math. Keep practicing, and soon these strategies will become second nature.
By the way, if you need some more worksheets to practice ADD & SUBTRACT BY 7-9 ONES WITHIN 100 USING MENTAL STRATEGIES, I have a free resource for you! Inside include a blank template so that you can insert any number you want!
Just drop your email below and it will be sent to your inbox directly!
FAQs
What is the compensation strategy in math?
The compensation strategy in math involves adjusting numbers to make calculations easier and then compensating for the adjustments to find the correct answer.
How can I improve my compensation strategy skills?
Practice regularly with different types of problems, use visual aids, and break down complex problems into simpler parts. You can use this worksheet for more practice: Math Compensation Strategy For Addition And Subtraction Within 100 Worksheets
Can compensation strategy math help in real-life situations?
Absolutely! It can help with quick mental calculations in everyday tasks like shopping, cooking, and budgeting.
How long does it take to master compensation strategy math?
The time it takes to master compensation strategy math varies depending on practice frequency and individual learning pace, but regular practice can lead to significant improvement in a few months.
Here are some pins that you can save to bookmark this post. Save them to your Pinterest Board now! ↓